A function \(f:D\rightarrow \mathbb{R}\) (with \(D\) a subset of \(\mathbb{R}^n\)) is called Lipschitz continuous if there exists a constant \(\lambda \geq 0\) such that for all \(\mathbf{x}, \mathbf{y}\in D\) the following inequality holds:
\[\vert f(\mathbf{x}) - f(\mathbf{y})\vert \leq \lambda \vert\vert\mathbf{x} - \mathbf{y}\vert\vert\]Let us denote smallest such \(\lambda\) as \(\lambda^*\) and call it the Lipschitz constant.
Importance for sphere tracing
As explained by J. C. Hart, sphere tracing requires Lipschitz continuity to work correctly. Morever, the associated \(\lambda^*\) has to be smaller than or equal to \(1\). If this is not the case, we cannot take \(f(\mathbf{x})\) as the step size along the ray without the risk to “overshoot” the surface.
To see this, recall that \(f_S\) is a signed distance bound of \(S\) if and only if for all \(\mathbf{x}\in\mathbb{R}^3\) we have
\[\vert f_S(\mathbf{x})\vert\leq \min_{\mathbf{y}\in f_S^{-1}(0)}\vert\vert\mathbf{x} - \mathbf{y}\vert\vert_2\]where \(f_S^{-1}(0)=\{\mathbf{z}: f_S(\mathbf{z})=0\}\). If we assume that \(f_S\) is Lipschitz continuous with Lipschitz constant \(\lambda^*\), then for any point \(\mathbf{x}\) and \(\mathbf{z}\) such that
\[\vert\vert\mathbf{x} - \mathbf{z}\vert\vert_2= \min_{\mathbf{y}\in f_S^{-1}(0)}\vert\vert\mathbf{x} - \mathbf{y}\vert\vert_2\]we have
\[\vert f(\mathbf{x})\vert\leq \lambda^*\vert\vert\mathbf{x} - \mathbf{z}\vert\vert_2= \lambda^*\min_{\mathbf{y}\in f_S^{-1}(0)}\vert\vert\mathbf{x} - \mathbf{y}\vert\vert_2\]Thus, to mitigate overestimating the distance, in which case sphere tracing can “overshoot” the surface and penetrate the shape, we must have \(\lambda^*\leq 1\). This is a sufficient condition to avoid the mentioned problem.
If \(\lambda^*\) is greater than \(1\), we can replace \(f_S\) by \(f_S/\lambda^*\). The resulting function has an optimal Lipschitz constant, \(1\).
In the context of the LambdaCAD tool, we can get holes in the generated mesh if \(\lambda^* > 1\). This is demonstrated in the next section.
An illustrative example
Let us consider a shape defined by a implicit function \(F(x, y, z) = x^2 + y^2 + z^2 - 4 = 0\). It is not hard to see that this is a sphere of radius \(2\) centered at the origin. The function \(F\) is not Lipschitz continuous on \(\mathbb{R}^3\) since it’s derivative is not bounded (more on that later). However, if we constrain ourselves to a region \([-2, +2]^3\), then we can construct a signed distance bound \(f(x, y, z)=F(x, y, z)/\lambda\) for \(\lambda=4\).
The following snippet is an implementation of \(f\) for https://nenadmarkus.com/lambda:
return function (x, y, z) {
const r = 2;
const lam = 4;
return (x*x + y*y + z*z - r*r)/lam;
};
Setting size=4 and resolution=100 produces the following mesh:

The above representation looks as expected.
However, if we change \(\lambda\) from \(4\) to \(3\) (set lam=3 in the code snippet), we get holes in the model (some triangles are missing):
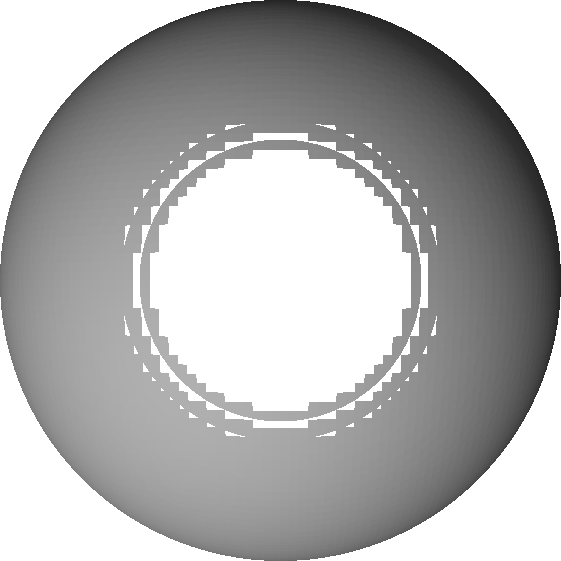
This is due to sphere tracing occasionally “missing” the surface of the shape and immediately “jumping” in its interior. A consequence of overestimating some distances because of wrong \(\lambda\).
Useful properties related to Lipschitz continuity
We assume that \(f\) and \(g\) are Lipschitz continuous with associated constants \(\lambda_f\) and \(\lambda_g\), respectively.
- The sum \(f+g\) is Lipschitz continuous:
- If \(\vert f\vert\) and \(\vert g\vert\) are bounded by some constant \(M\), then the product \(fg\) is Lipschitz continuous:
- A continuous and differentiable function \(f\) is Lipschitz if and only if its derivative \(f'\) is bounded. To prove the first implication, we assume that \(f\) is Lipschitz continuos and work with the definition of the derivative:
The other implication follows from the mean value theorem. There exists \(c\) between \(x\) and \(y\) such that
\[\vert f(x) - f(y)\vert\leq \vert f'(c)\vert\cdot\vert x - y\vert\]If we assume that \(\vert f'(c)\vert\leq M\), then it follows that \(f\) is Lipschitz continuous.
- The composition \(f\circ g\) is Lipschitz continuous:
Resources
- J. C. Hart. Sphere tracing: A geometric method for the antialiased ray tracing of implicit surfaces. The Visual Computer, 1994.
- https://en.wikipedia.org/wiki/Lipschitz_continuity
- https://math.stackexchange.com/questions/2823582/sum-of-lipschitz-functions
- https://math.stackexchange.com/questions/1028974/sum-and-product-of-lipschitz-functions
- https://math.stackexchange.com/questions/1165431/is-a-function-lipschitz-if-and-only-if-its-derivative-is-bounded